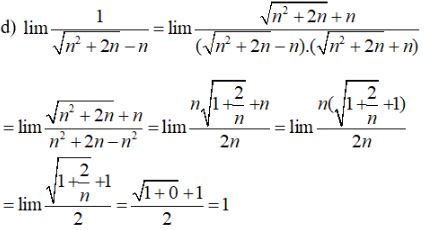Câu 17 trang 226 SGK Đại số và Giải tích 11 Nâng cao

LG a

\(\lim \sqrt {3{n^4} - 10n + 12} \)

Lời giải chi tiết:

\(\lim \sqrt {3{n^4} - 10n + 12}  \) \(= \lim {n^2}.\sqrt {3 - {{10} \over {{n^3}}} + {{12} \over {{n^4}}}}  \) \(=  + \infty \)

Vì 

\(\left\{ \begin{array}{l}
\lim {n^2} = + \infty \\
\lim \sqrt {3 - \frac{{10}}{{{n^3}}} + \frac{{12}}{{{n^4}}}} = \sqrt 3 > 0
\end{array} \right.\)

LG b

\(\lim \left( {{{2.3}^n} - {{5.4}^n}} \right)\)

Lời giải chi tiết:

\(\lim \left( {{{2.3}^n} - {{5.4}^n}} \right) \) \(= \lim {4^n}\left[ {2{{\left( {{3 \over 4}} \right)}^n} - 5} \right] =  - \infty \)

Vì 

\(\left\{ \begin{array}{l}
\lim {4^n} = + \infty \\
\lim \left( {2.{{\left( {\frac{3}{4}} \right)}^n} - 5} \right) = - 5 < 0
\end{array} \right.\)

LG c

\(\lim \left( {\sqrt {{n^4} + {n^2} + 1}  - {n^2}} \right)\)

Lời giải chi tiết:

\(\eqalign{  & \lim \left( {\sqrt {{n^4} + {n^2} + 1}  - {n^2}} \right) \cr& = \lim \frac{{\left( {\sqrt {{n^4} + {n^2} + 1}  - {n^2}} \right)\left( {\sqrt {{n^4} + {n^2} + 1}  + {n^2}} \right)}}{{\sqrt {{n^4} + {n^2} + 1}  + {n^2}}} \cr &= \lim \frac{{{n^4} + {n^2} + 1 - {n^4}}}{{\sqrt {{n^4} + {n^2} + 1}  + {n^2}}}\cr &= \lim {{{n^2} + 1} \over {\sqrt {{n^4} + {n^2} + 1}  + {n^2}}}  \cr  &  = \lim \frac{{{n^2} + 1}}{{\sqrt {{n^4}\left( {1 + \frac{1}{{{n^2}}} + \frac{1}{{{n^4}}}} \right)}  + {n^2}}} \cr  &  = \lim \frac{{{n^2}\left( {1 + \frac{1}{{{n^2}}}} \right)}}{{{n^2}\sqrt {1 + \frac{1}{{{n^2}}} + \frac{1}{{{n^4}}}}  + {n^2}}} \cr  &  = \lim \frac{{{n^2}\left( {1 + \frac{1}{{{n^2}}}} \right)}}{{{n^2}\left( {\sqrt {1 + \frac{1}{{{n^2}}} + \frac{1}{{{n^4}}}}  + 1} \right)}}\cr & = \lim {{1 + {1 \over {{n^2}}}} \over {\sqrt {1 + {1 \over {{n^2}}} + {1 \over {{n^4}}}}  + 1}} \cr & = \frac{{1 + 0}}{{\sqrt {1 + 0 + 0}  + 1}}= {1 \over 2} \cr} \)

LG d

\(\lim {1 \over {\sqrt {{n^2} + 2n}  - n}}\) 

Lời giải chi tiết:

 HocTot.Nam.Name.Vn