Phương pháp giải:

Phương pháp:

Định nghĩa đạo hàm: Nếu hàm số $f\left( x \right)$ xác định tại ${x_0}$ và tồn tại giới hạn $\mathop {\lim }\limits_{x \to {x_0}} \dfrac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ thì hàm số $f\left( x \right)$ có đạo hàm tại ${x_0}$.

Định nghĩa hàm số liên tục tại một điểm: Nếu hàm số $f\left( x \right)$ xác định tại ${x_0}$ và $\mathop {\lim }\limits_{x \to {x_0}} f\left( x \right) = f\left( {{x_0}} \right)$ thì hàm số liên tục tại ${x_0}$.

Lời giải chi tiết:

Cách giải:

Dựa vào định nghĩa đạo hàm, ta có kết quả:

Nếu hàm số $y = f\left( x \right)$ có đạo hàm tại ${x_0}$ thì tồn tại giới hạn $L = \mathop {\lim }\limits_{x \to {x_0}} \dfrac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$.

Do đó $\mathop {\lim }\limits_{x \to {x_0}} f\left( x \right) = f\left( {{x_0}} \right)$ vì nếu $\mathop {\lim }\limits_{x \to {x_0}} f\left( x \right) \ne f\left( {{x_0}} \right)$ thì $\mathop {\lim }\limits_{x \to {x_0}} \dfrac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}} =  \pm \infty$

Do đó hàm số liên tục tại điểm $x = {x_0}$.

Chọn đáp án D

Phương pháp giải:

Phương pháp. Sử dụng định nghĩa, công thức đạo hàm cơ bản để tính trực tiếp đạo hàm và kết luận.

Lời giải chi tiết:

Lời giải chi tiết.

Ta có $x>1$ thì $f\left( x \right)={{x}^{2}}+1$ nên $f'\left( x \right)=2x\Rightarrow f'\left( 2 \right)=2.2=4.$

Đáp án D đúng.

Tương tự ta có $f'\left( 0 \right)=2$

đáp án C đúng.

Ta kiểm tra xem $f$ có đạo hàm tại ${{x}_{0}}=1$ hay không?

Ta có $\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{\left( {{x}^{2}}+1 \right)-2}{x-1}=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{{{x}^{2}}-1}{x-1}=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\left( x+1 \right)=2.$

Tương tự ta có $\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{2x-2}{x-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{2\left( x-1 \right)}{x-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,2=2.$

Như vậy $\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=2.$

Do đó $f'\left( 1 \right)=2.$

Đáp án A đúng.

Chọn đáp án B.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

TXĐ: $D=\left[ -1;+\infty  \right)$ 

$f'\left( 1 \right)=\underset{x\to 1}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to 1}{\mathop{\lim }}\,\frac{\sqrt{x+1}-\sqrt{2}}{x-1}=\underset{x\to 1}{\mathop{\lim }}\,\frac{x+1-2}{\left( x-1 \right)\left( \sqrt{x+1}+\sqrt{2} \right)}=\underset{x\to 1}{\mathop{\lim }}\,\frac{1}{\sqrt{x+1}+\sqrt{2}}=\frac{1}{2\sqrt{2}}=\frac{\sqrt{2}}{4}$

Chọn A.

Phương pháp giải:

Xét tính đúng sai ở từng bước.

Lời giải chi tiết:

Bài giải trên hoàn toàn đúng.

Chọn D.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$f'\left( 0 \right)=\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}=\underset{x\to 0}{\mathop{\lim }}\,\frac{3-\sqrt{4-x}-1}{x}=\underset{x\to 0}{\mathop{\lim }}\,\frac{2-\sqrt{4-x}}{x}=\underset{x\to 0}{\mathop{\lim }}\,\frac{4-4+x}{x\left( 2+\sqrt{4-x} \right)}=\underset{x\to 0}{\mathop{\lim }}\,\frac{1}{2+\sqrt{4-x}}=\frac{1}{4}$

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{\sqrt x  - 1}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{1}{{\sqrt x  + 1}} = \frac{1}{2}\\\mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{{x^2} - 1}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ - }} \left( {x + 1} \right) = 2\\ \Rightarrow \mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} \ne \mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}\end{array}$

Vậy không tồn tại đạo hàm của hàm số tại x = 1.

Chọn D.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Ta có: $\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}=\underset{x\to 0}{\mathop{\lim }}\,\frac{\sqrt{x}}{{{x}^{2}}}=\underset{x\to 0}{\mathop{\lim }}\,\frac{1}{x\sqrt{x}}=+\infty \Rightarrow$ Hàm số không có đạo hàm tại x = 0.

Chọn B.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{2x + 3 - 5}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{2x - 2}}{{x - 1}} = 2\\\mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}   =  \mathop {\lim }\limits_{x \to {1^ - }} \frac{{\frac{{{x^3} + 2{x^2} - 7x + 4}}{{x - 1}} - 5}}{{x - 1}}\\ = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{{x^3} + 2{x^2} - 12x + 9}}{({x - 1})^2} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{\left( {x - 1} \right)\left( {{x^2} + 3x - 9} \right)}}{({x - 1})^2}\\ = \mathop {\lim }\limits_{x \to {1^ - }} {{ {{x^2} + 3x - 9} }\over{x-1} }=  +\infty.\\ \Rightarrow \mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} \ne \mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}\end{array}$

Vậy hàm số không tồn tại đạo hàm tại x = 1.

Chọn D. 

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại)

Lời giải chi tiết:

$f'\left( 0 \right)=\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}=\underset{x\to 0}{\mathop{\lim }}\,\frac{\frac{\sqrt{{{x}^{2}}+1}-1}{x}}{x}=\underset{x\to 0}{\mathop{\lim }}\,\frac{\sqrt{{{x}^{2}}+1}-1}{{{x}^{2}}}=\underset{x\to 0}{\mathop{\lim }}\,\frac{{{x}^{2}}+1-1}{{{x}^{2}}\left( \sqrt{{{x}^{2}}+1}+1 \right)}=\underset{x\to 0}{\mathop{\lim }}\,\frac{1}{\sqrt{{{x}^{2}}+1}+1}=\frac{1}{2}$

Chọn A.

Phương pháp giải:

Suy luận từ công thức tính đạo hàm của hàm số tại một điểm bằng định nghĩa.

Lời giải chi tiết:

(I) hiển nhiên đúng.

(II) sai.

Ví dụ: Xét hàm số $f\left( x \right)=\left| x \right|$ ta có

$\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,=\left| {{x}_{0}} \right|=f\left( {{x}_{0}} \right)\Rightarrow$ Hàm số liên tục tại trên R. Tuy nhiên hàm số không có đạo hàm tại x = 0

$\begin{array}{l}f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{\left| x \right| - 0}}{{x - 0}} = \mathop {\lim }\limits_{x \to 0} \frac{{\left| x \right|}}{x}\\\left\{ \begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{\left| x \right|}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{x}{x} = 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{\left| x \right|}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{ - x}}{x} =  - 1\end{array} \right. \Rightarrow \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\left| x \right|}}{x} \ne \mathop {\lim }\limits_{x \to {0^ - }} \frac{{\left| x \right|}}{x}\end{array}$

Không tồn tại đạo hàm của hàm số tại x = 0. 

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\left\{ \begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{{x^2}}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} x = 0\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{ - {x^2}}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \left( { - x} \right) = 0\end{array} \right. \Rightarrow \mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = 0 \Rightarrow$ Hàm số $y=f\left( x \right)$ có đạo hàm tại x = 0.

$\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{g\left( x \right)-g\left( 0 \right)}{x-0}=\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{\sqrt{x}}{x}=+\infty \Rightarrow$ Hàm số $y=g\left( x \right)$ không có đạo hàm tại x = 0.

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Để hàm số có đạo hàm tại x = 0, trước hết hàm số phải liên tục tại x = 0.

Ta có :

$\begin{array}{l}\mathop {\lim }\limits_{x \to 0} f\left( x \right) = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt[3]{{4{x^2} + 8}} - \sqrt {8{x^2} + 4} }}{{{x^2}}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt[3]{{4{x^2} + 8}} - 2}}{{{x^2}}} - \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt {8{x^2} + 4}  - 2}}{{{x^2}}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{4{x^2}}}{{{x^2}\left( {{{\sqrt[3]{{4{x^2} + 8}}}^2} + 2\sqrt[3]{{4{x^2} + 8}} + 4} \right)}} - \mathop {\lim }\limits_{x \to 0} \frac{{8{x^2}}}{{{x^2}\left( {\sqrt {8{x^2} + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{4}{{{{\sqrt[3]{{4{x^2} + 8}}}^2} + 2\sqrt[3]{{4{x^2} + 8}} + 4}} - \mathop {\lim }\limits_{x \to 0} \frac{8}{{\sqrt {8{x^2} + 4}  + 2}} = \frac{1}{3} - 2 =  - \frac{5}{3}\end{array}$

$f\left( 0 \right) = 0$

$\Rightarrow \mathop {\lim }\limits_{x \to 0} f\left( x \right) \ne f\left( 0 \right)$, do đó hàm số không liên tục tại $x = 0$.

Vậy hàm số không có đạo hàm tại $x = 0$.

Chọn D.

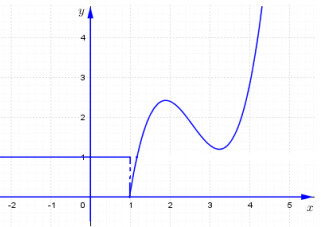
Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Dựa vào đồ thị hàm số ta thấy $\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,f\left( x \right)=1,\,\,\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,f\left( x \right)=0\Rightarrow \underset{x\to {{1}^{-}}}{\mathop{\lim }}\,f\left( x \right)\ne \underset{x\to {{1}^{+}}}{\mathop{\lim }}\,f\left( x \right)\Rightarrow$ Không tồn tại $\underset{x\to 1}{\mathop{\lim }}\,f\left( x \right)$, hàm số không liên tục tại x = 1.

Vậy hàm số không có tại hàm tại x = 1

Chọn B.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Ta có: $f'\left( 1 \right)=\underset{x\to 1}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to 1}{\mathop{\lim }}\,\frac{\frac{{{x}^{3}}-4{{x}^{2}}+3x}{{{x}^{2}}-3x+2}}{x-1}=\underset{x\to 1}{\mathop{\lim }}\,\frac{x\left( x-3 \right)\left( x-1 \right)}{{{\left( x-1 \right)}^{2}}\left( x-2 \right)}=\underset{x\to 1}{\mathop{\lim }}\,\frac{x\left( x-3 \right)}{\left( x-1 \right)\left( x-2 \right)}=-\infty$

Vậy hàm số không có đạo hàm tại x = 1.

Chọn D.

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$f'\left( -1 \right)=\underset{x\to \left( -1 \right)}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( -1 \right)}{x+1}$

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ + }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ + }} \frac{{\frac{{{x^2} + x + 1}}{x} + 1}}{{x + 1}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ + }} \frac{{{x^2} + 2x + 1}}{{x\left( {x + 1} \right)}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ + }} \frac{{x + 1}}{x} = 0\\\mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ - }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ - }} \frac{{\frac{{{x^2} - x - 1}}{x} + 1}}{{x + 1}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ - }} \frac{{{x^2} - 1}}{{x\left( {x + 1} \right)}} = \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ - }} \frac{{x - 1}}{x} = 2\\ \Rightarrow \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ + }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}} \ne \mathop {\lim }\limits_{x \to {{\left( { - 1} \right)}^ - }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}}\end{array}$

Do đó không tồn tại  $\underset{x\to \left( -1 \right)}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( -1 \right)}{x+1}$, vậy không tồn tại đạo hàm của hàm số tại ${{x}_{0}}=-1$.

Chọn D.

Phương pháp giải:

+) Hàm số liên tục tại $x={{x}_{0}}\Leftrightarrow \underset{x\to x_{0}^{+}}{\mathop{\lim }}\,f\left( x \right)=\underset{x\to x_{0}^{-}}{\mathop{\lim }}\,f\left( x \right)=f\left( 0 \right)$

+) Hàm số có đạo hàm tại $x={{x}_{0}}\Leftrightarrow f'\left( {{x}_{0}} \right)=\underset{x\to x_{0}^{+}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}=\underset{x\to x_{0}^{-}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$

Lời giải chi tiết:

Ta có: $y = \frac{{\left| x \right|}}{{x + 1}} = \left\{ \begin{array}{l}\frac{x}{{x + 1}}\,\,\,khi\,x \ge 0\\\frac{{ - x}}{{x + 1}}\,\,\,khi\,\,x < 0\end{array} \right.$

Ta có $\left\{ \begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \frac{x}{{x + 1}} = 0 = f\left( 0 \right)\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{ - x}}{{x + 1}} = 0\end{array} \right. \Rightarrow \mathop {\lim }\limits_{x \to x_0^ + } f\left( x \right) = \mathop {\lim }\limits_{x \to x_0^ - } f\left( x \right) = f\left( 0 \right) = 0 \Rightarrow$ Hàm số liên tục tại x = 0.

$f'\left( 0 \right)=\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}$ 

Ta có:

$\left\{ \begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\frac{x}{{x + 1}} - 0}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{1}{{x + 1}} = 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{\frac{{ - x}}{{x + 1}} - 0}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{ - 1}}{{x + 1}} =  - 1\end{array} \right. \Rightarrow x = {x_0} \Rightarrow \mathop {\lim }\limits_{x \to x_0^ + } \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}} \ne \mathop {\lim }\limits_{x \to x_0^ - } \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}} \Rightarrow$ Hàm số không tồn tại đạo hàm tại x = 0.

Chọn B.

Phương pháp giải:

+) Để hàm số có đạo hàm tại x = 1 thì hàm số phải liên tục tại x = 1.

+) Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Để hàm số có đạo hàm của hàm số tại điểm x = 1 thì trước hết hàm số phải liên tục tại x = 1, tức là $\underset{x\to 1}{\mathop{\lim }}\,f\left( x \right)=f\left( 1 \right)\Leftrightarrow \underset{x\to 1}{\mathop{\lim }}\,\frac{{{x}^{2}}-1}{x+1}=a\Leftrightarrow \underset{x\to 1}{\mathop{\lim }}\,\left( x+1 \right)=a\Leftrightarrow 2=a$

Khi đó hàm số có dạng: $f\left( x \right) = \left\{ \begin{array}{l}\frac{{{x^2} - 1}}{{x - 1}}\,\,khi\,\,x \ne 1\\2\,\,\,\,\,\,\,\,\,\,\,\,\,khi\,\,x = 1\end{array} \right.$

$\Rightarrow f'\left( 1 \right)=\underset{x\to 1}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to 1}{\mathop{\lim }}\,\frac{\frac{{{x}^{2}}-1}{x-1}-2}{x-1}\underset{x\to 1}{\mathop{\lim }}\,\frac{\left( x+1 \right)\left( x-1 \right)-2\left( x-1 \right)}{{{\left( x-1 \right)}^{2}}}=\underset{x\to 1}{\mathop{\lim }}\,\frac{x+1-2}{x-1}=1$

Vậy a = 2.

Chọn B.

Phương pháp giải:

+) Trước hết hàm số liên tục tại x = 0.

+) Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là

$f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}\Leftrightarrow f'\left( {{x}_{0}} \right)=\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}$

Lời giải chi tiết:

Trước tiên hàm số phải liên tục tại x = 0.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{{x^2} + 1}}{{x + 1}} = 1\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \left( {ax + b} \right) = b = f\left( 0 \right)\end{array}$

Để hàm số liên tục tại x = 0 thì $\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,f\left( x \right)=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,f\left( x \right)=f\left( 0 \right)\Leftrightarrow b=1$

Khi đó ta có $f'\left( 0 \right)=\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}$

Ta có

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\frac{{{x^2} + 1}}{{x + 1}} - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{{x^2} - x}}{{x\left( {x + 1} \right)}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{x - 1}}{{x + 1}} =  - 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\left( {ax + 1} \right) - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} a = a\end{array}$

Để hàm số có đạo hàm tại x = 0 thì  $\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x}\Leftrightarrow a=-1$

Vậy $a=-1,b=1$.

Chọn D.

Phương pháp giải:

+) Tìm điều kiện để hàm số liên tục tại x = 1: $\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,f\left( x \right)=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,f\left( x \right)=f\left( 1 \right)$

+) Tìm điều kiện để hàm số có đạo hàm tại x = 1: $f'\left( 1 \right)=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}$

Lời giải chi tiết:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ + }} \left( {a{x^2} + bx} \right) = a + b = f\left( 1 \right)\\\mathop {\lim }\limits_{x \to {1^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ - }} \left( {2x - 1} \right) = 1\end{array}$

Để hàm số liên tục tại x = 1 thì $\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,f\left( x \right)=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,f\left( x \right)=f\left( 1 \right)\Leftrightarrow a+b=1\,\,\,\left( 1 \right)$

Khi đó ta có: $f'\left( 1 \right)=\underset{x\to 1}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}$

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{a{x^2} + bx - \left( {a + b} \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{a\left( {{x^2} - 1} \right) + b\left( {x - 1} \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \left[ {a\left( {x + 1} \right) + b} \right] = 2a + b\\\mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{2x - 1 - \left( {a + b} \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{2x - 2}}{{x - 1}} = 2\end{array}$

Để hàm số có đạo hàm tại x = 1 thì $f'\left( 1 \right)=\underset{x\to {{1}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}=\underset{x\to {{1}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 1 \right)}{x-1}\Leftrightarrow 2a+b=2\,\,\,\left( 2 \right)$

Từ (1) và (2) ta có hệ: $\left\{ \begin{array}{l}a + b = 1\\2a + b = 2\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}a = 1\\b = 0\end{array} \right.$

Chọn C.

Phương pháp giải:

Để hàm số có đạo hàm tại x₀ thì hàm số liên tục tại x₀, điều ngược lại chưa chắc đúng.

Lời giải chi tiết:

Một hàm số liên tục tại x₀ chưa chắc có đạo hàm tại điểm đó, hơn nữa

$\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}=\underset{x\to 0}{\mathop{\lim }}\,\frac{x\sin \frac{\pi }{x}-0}{x}=\underset{x\to 0}{\mathop{\lim }}\,\sin \frac{\pi }{x}=+\infty \Rightarrow$ Hàm số không có đạo hàm tại x = 0. Lập luận trên sai từ bước 4.

Chọn D.  

Phương pháp giải:

Đạo hàm của hàm số $y=f\left( x \right)$ tại điểm $x={{x}_{0}}$ là $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$

Lời giải chi tiết:

$\begin{array}{l}f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to 0} \frac{{x\left( {x - 1} \right)\left( {x - 2} \right)...\left( {x - 1000} \right) - 0}}{x}\\ = \mathop {\lim }\limits_{x \to 0} \left( {x - 1} \right)\left( {x - 2} \right)...\left( {x - 1000} \right) = \mathop {\lim }\limits_{x \to 0} \left( { - 1} \right)\left( { - 2} \right)\left( { - 3} \right)...\left( { - 1000} \right) = {\left( { - 1} \right)^{1000}}.1000! = 1000!\end{array}$

Chọn B.

Phương pháp giải:

+) Trước hết, tìm điều kiện để hàm số liên tục tại x = 0.

+) Sử dụng công thức tính đạo hàm bằng định nghĩa.

+) Hàm số có đạo hàm tại x = 0 $\Leftrightarrow \underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}\Leftrightarrow a=1.$ Sử dụng công thức $\underset{x\to 0}{\mathop{\lim }}\,\frac{\sin x}{x}=1$ .

Lời giải chi tiết:

Để hàm số có đạo hàm tại x = 1 thì trước hết hàm số phải liên tục tại x = 1.

Ta có: $f\left( 0 \right)=1$

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \left( {a{x^2} + bx + 1} \right) = 1 = f\left( 0 \right)\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \left( {a\sin x + b\cos x} \right) = b\end{array}$

Để hàm số liên tục tại x = 1 thì $\underset{x\to {{0}^{+}}}{\mathop{\lim }}\,f\left( x \right)=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,f\left( x \right)=f\left( 0 \right)\Leftrightarrow b=1$

Khi đó ta có: $f'\left( 0 \right)=\underset{x\to 0}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}$

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{a{x^2} + x + 1 - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \left( {ax + 1} \right) = 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{a\sin x + \cos x - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{2a\sin \frac{x}{2}\cos \frac{x}{2} - 2{{\sin }^2}\frac{x}{2}}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{\sin \frac{x}{2}}}{{\frac{x}{2}}}\mathop {\lim }\limits_{x \to {0^ - }} \left( {a\cos \frac{x}{2} - 2\sin \frac{x}{2}} \right) = a\end{array}$Để tồn tại $f'\left( 0 \right)\Leftrightarrow \underset{x\to {{0}^{+}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}=\underset{x\to {{0}^{-}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 0 \right)}{x-0}\Leftrightarrow a=1.$

Chọn A.

Phương pháp giải:

Sử dụng công thức đạo hàm của hàm số hợp $\left( \sqrt{u} \right)'=\frac{u'}{2\sqrt{u}}$

Lời giải chi tiết:

$\begin{array}{l}f\left( x \right) = \left| x \right|\\\left\{ \begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \frac{x}{x} = 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \frac{{ - x}}{x} =  - 1\end{array} \right. \Rightarrow \mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} \ne \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}\end{array}$  

Do đó không tồn tại $f'\left( 0 \right)$ của hàm số trên.

Chọn D.

Phương pháp giải:

Sử dụng công thức tính đạo hàm bằng định nghĩa $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} {{f\left( x \right) - f\left( {{x_0}} \right)} \over {x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\mathop {\lim }\limits_{x \to 2} {{f\left( x \right) - f\left( 2 \right)} \over {x - 2}} = 3 \Rightarrow f'\left( 2 \right) = 3$

Chọn B.

Phương pháp giải:

Sử dụng công thức tính đạo hàm bằng định nghĩa $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} {{f\left( x \right) - f\left( {{x_0}} \right)} \over {x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\mathop {\lim }\limits_{x \to  - 1} {{f\left( x \right) - f\left( { - 1} \right)} \over {x + 1}} = f'\left( { - 1} \right)$.

Chọn C.

Phương pháp giải:

Sử dụng công thức tính đạo hàm bằng định nghĩa:  $f'\left( {{x}_{0}} \right)=\underset{x\to {{x}_{0}}}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( {{x}_{0}} \right)}{x-{{x}_{0}}}$ (nếu tồn tại giới hạn).

Lời giải chi tiết:

Ta có: $f'\left( 6 \right)=\underset{x\to 6}{\mathop{\lim }}\,\frac{f\left( x \right)-f\left( 6 \right)}{x-6}=2$

Chọn A.

Phương pháp giải:

Sử dụng định nghĩa tính đạo hàm của một hàm số.

Lời giải chi tiết:

Chọn B.

Phương pháp giải:

+) Kiểm tra tính liên tục của hàm số tại $x = 1$.

+) Nếu hàm số liên tục tại $x = 1$, sử dụng công thức tính đạo hàm bằng định nghĩa: $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \dfrac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$.

Lời giải chi tiết:

Trước hết ta xét tính liên tục của hàm số tại $x = 1$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to 1} f\left( x \right) = \mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {3x + 1}  - 2x}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {\sqrt {3x + 1}  - 2x} \right)\left( {\sqrt {3x + 1}  + 2x} \right)}}{{\left( {x - 1} \right)\left( {\sqrt {3x + 1}  + 2x} \right)}}\\ = \mathop {\lim }\limits_{x \to 1} \dfrac{{3x + 1 - 4{x^2}}}{{\left( {x - 1} \right)\left( {\sqrt {3x + 1}  + 2x} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{ - \left( {x - 1} \right)\left( {4x + 1} \right)}}{{\left( {x - 1} \right)\left( {\sqrt {3x + 1}  + 2x} \right)}}\\ = \mathop {\lim }\limits_{x \to 1} \dfrac{{ - 4x - 1}}{{\sqrt {3x + 1}  + 2x}} = \dfrac{{ - 4 - 1}}{{\sqrt 4  + 2}} = \dfrac{{ - 5}}{4} = f\left( 1 \right)\end{array}$

$\Rightarrow$ Hàm số liên tục tại $x = 1$.

Tính $f'\left( 1 \right)$.

$\begin{array}{l} \Rightarrow f'\left( 1 \right) = \mathop {\lim }\limits_{x \to 1} \dfrac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \dfrac{{\dfrac{{\sqrt {3x + 1}  - 2x}}{{x - 1}} + \dfrac{5}{4}}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{4\sqrt {3x + 1}  - 8x + 5x - 5}}{{4{{\left( {x - 1} \right)}^2}}}\\ = \mathop {\lim }\limits_{x \to 1} \dfrac{{4\sqrt {3x + 1}  - 3x - 5}}{{4{{\left( {x - 1} \right)}^2}}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {4\sqrt {3x + 1}  - 3x - 5} \right)\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}}{{4{{\left( {x - 1} \right)}^2}\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}}\\ = \mathop {\lim }\limits_{x \to 1} \dfrac{{16\left( {3x + 1} \right) - \left( {9{x^2} + 30x + 25} \right)}}{{4{{\left( {x - 1} \right)}^2}\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{ - 9{x^2} + 18x - 9}}{{4{{\left( {x - 1} \right)}^2}\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}}\\ = \mathop {\lim }\limits_{x \to 1} \dfrac{{ - 9{{\left( {x - 1} \right)}^2}}}{{4{{\left( {x - 1} \right)}^2}\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{ - 9}}{{4\left( {4\sqrt {3x + 1}  + 3x + 5} \right)}} = \dfrac{{ - 9}}{{64}}\end{array}$

Chọn C.

Phương pháp giải:

Lời giải chi tiết:

Nếu hàm số $y = f\left( x \right)$ có đạo hàm tại điểm $x = {x_0}$ thì $y = f\left( x \right)$ liên tục tại điểm đó và nếu hàm số $y = f\left( x \right)$ gián đoạn tại điểm $x = {x_0}$ thì chắc chắn $y = f\left( x \right)$ không có đạo hàm tại điểm đó là 2 mệnh đề đúng.

Chọn B.

Phương pháp giải:

Sử dụng công thức tính đạo hàm bằng định nghĩa.

Lời giải chi tiết:

Tính$f'\left( {{x_0}} \right)$ bằng định nghĩa ta cần tính  $\mathop {\lim }\limits_{\Delta x \to 0} \frac{{\Delta y}}{{\Delta x}}$.

Chọn B.

Phương pháp giải:

Số gia $\Delta y$ của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ ứng với số gia $\Delta x$ là $\Delta y = f\left( {{x_0} + \Delta x} \right) - f\left( {{x_0}} \right)$. 

Lời giải chi tiết:

Đặt $y = {x^2} = f\left( x \right)$ ta có:

$\begin{array}{l}\Delta y = f\left( {{x_0} + \Delta x} \right) - f\left( {{x_0}} \right) = f\left( {3 - 1} \right) - f\left( 3 \right)\\\,\,\,\,\,\,\, = f\left( 2 \right) - f\left( 3 \right) = {2^2} - {3^2} =  - 5\end{array}$

Chọn C.

Phương pháp giải:

Lời giải chi tiết:

Hệ thức đúng là: $f'\left( 1 \right) = \mathop {\lim }\limits_{x \to 1} \dfrac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}.$

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

TXĐ: $D = \left[ { - 1; + \infty } \right)$ 

$\begin{array}{l}f'\left( 1 \right) = \mathop {\lim }\limits_{x \to 1} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\sqrt {x + 1}  - \sqrt 2 }}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{x + 1 - 2}}{{\left( {x - 1} \right)\left( {\sqrt {x + 1}  + \sqrt 2 } \right)}}\\ = \mathop {\lim }\limits_{x \to 1} \frac{1}{{\sqrt {x + 1}  + \sqrt 2 }} = \frac{1}{{2\sqrt 2 }} = \frac{{\sqrt 2 }}{4}\end{array}$

Chọn A.

Phương pháp giải:

Xét tính đúng sai ở từng bước.

Lời giải chi tiết:

Bài giải trên hoàn toàn đúng.

Chọn D.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\begin{array}{l}f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to 0} \frac{{3 - \sqrt {4 - x}  - 1}}{x} = \mathop {\lim }\limits_{x \to 0} \frac{{2 - \sqrt {4 - x} }}{x}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{4 - 4 + x}}{{x\left( {2 + \sqrt {4 - x} } \right)}} = \mathop {\lim }\limits_{x \to 0} \frac{1}{{2 + \sqrt {4 - x} }} = \frac{1}{4}\end{array}$

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{2x + 3 - 5}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{2x - 2}}{{x - 1}} = 2\\\mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{\frac{{{x^3} + 2{x^2} - 7x + 4}}{{x - 1}} - 5}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{{x^3} + 2{x^2} - 12x + 9}}{{{{\left( {x - 1} \right)}^2}}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{\left( {x - 1} \right)\left( {{x^2} + 3x - 9} \right)}}{{{{\left( {x - 1} \right)}^2}}} = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{{x^2} + 3x - 9}}{{x - 1}} =  + \infty \\ \Rightarrow \mathop {\lim }\limits_{x \to {1^ + }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} \ne \mathop {\lim }\limits_{x \to {1^ - }} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}\end{array}$

Vậy hàm số không tồn tại đạo hàm tại x = 1.

Chọn D.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

$\begin{array}{l}f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{{\sqrt {{x^2} + 1}  - 1}}{x}}}{x} = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt {{x^2} + 1}  - 1}}{{{x^2}}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{{x^2} + 1 - 1}}{{{x^2}\left( {\sqrt {{x^2} + 1}  + 1} \right)}} = \mathop {\lim }\limits_{x \to 0} \frac{1}{{\sqrt {{x^2} + 1}  + 1}} = \frac{1}{2}\end{array}$

Chọn A.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Để hàm số có đạo hàm tại x = 0, trước hết hàm số phải liên tục tại x = 0.

$\begin{array}{l}\mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt[3]{{4{x^2} + 8}} - \sqrt {8{x^2} + 4} }}{{{x^2}}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt[3]{{4{x^2} + 8}} - 2}}{{{x^2}}} - \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt {8{x^2} + 4}  - 2}}{{{x^2}}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{{4{x^2}}}{{{x^2}\left( {{{\sqrt[3]{{4{x^2} + 8}}}^2} + 2\sqrt[3]{{4{x^2} + 8}} + 4} \right)}} - \mathop {\lim }\limits_{x \to 0} \frac{{8{x^2}}}{{{x^2}\left( {\sqrt {8{x^2} + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to 0} \frac{4}{{{{\sqrt[3]{{4{x^2} + 8}}}^2} + 2\sqrt[3]{{4{x^2} + 8}} + 4}} - \mathop {\lim }\limits_{x \to 0} \frac{8}{{\sqrt {8{x^2} + 4}  + 2}} = \frac{1}{3} - 2 =  - \frac{5}{3}\end{array}$

Chọn B.

Phương pháp giải:

Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là $f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}}$ (nếu tồn tại).

Lời giải chi tiết:

Ta có:

$f'\left( 1 \right) = \mathop {\lim }\limits_{x \to 1} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\frac{{{x^3} - 4{x^2} + 3x}}{{{x^2} - 3x + 2}}}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{x\left( {x - 3} \right)\left( {x - 1} \right)}}{{{{\left( {x - 1} \right)}^2}\left( {x - 2} \right)}} = \mathop {\lim }\limits_{x \to 1} \frac{{x\left( {x - 3} \right)}}{{\left( {x - 1} \right)\left( {x - 2} \right)}} =  - \infty$

Vậy hàm số không có đạo hàm tại x = 1.

Chọn D.

Phương pháp giải:

+) Trước hết hàm số liên tục tại x = 0.

+) Đạo hàm của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là

$f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{{f\left( x \right) - f\left( {{x_0}} \right)}}{{x - {x_0}}} \Leftrightarrow f'\left( {{x_0}} \right) = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x}$

Lời giải chi tiết:

Trước tiên hàm số phải liên tục tại x = 0.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{{x^2} + 1}}{{x + 1}} = 1\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \left( {ax + b} \right) = b = f\left( 0 \right)\end{array}$

Để hàm số liên tục tại x = 0 thì $\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = f\left( 0 \right) \Leftrightarrow b = 1$

Khi đó ta có $f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{x}$

Ta có

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\frac{{{x^2} + 1}}{{x + 1}} - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{{x^2} - x}}{{x\left( {x + 1} \right)}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{x - 1}}{{x + 1}} =  - 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{\left( {ax + 1} \right) - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} a = a\end{array}$

Để hàm số có đạo hàm tại x = 0 thì  $\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{x} \Leftrightarrow a =  - 1$

Vậy $a =  - 1,b = 1$.

Chọn D.

Phương pháp giải:

+) Trước hết, tìm điều kiện để hàm số liên tục tại x = 0.

+) Sử dụng công thức tính đạo hàm bằng định nghĩa.

+) Hàm số có đạo hàm tại  $x = 0 \Leftrightarrow \mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} \Leftrightarrow a = 1.$

Sử dụng công thức $\mathop {\lim }\limits_{x \to 0} \frac{{\sin x}}{x} = 1$ .

Lời giải chi tiết:

Để hàm số có đạo hàm tại x = 1 thì trước hết hàm số phải liên tục tại x = 1.

Ta có: $f\left( 0 \right) = 1$

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \left( {a{x^2} + bx + 1} \right) = 1 = f\left( 0 \right)\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \left( {a\sin x + b\cos x} \right) = b\end{array}$

Để hàm số liên tục tại x = 1 thì $\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = f\left( 0 \right) \Leftrightarrow b = 1$

Khi đó ta có: $f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}$

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{a{x^2} + x + 1 - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ + }} \left( {ax + 1} \right) = 1\\\mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ + }} \frac{{a\sin x + \cos x - 1}}{x} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{2a\sin \frac{x}{2}\cos \frac{x}{2} - 2{{\sin }^2}\frac{x}{2}}}{x}\\ = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{\sin \frac{x}{2}}}{{\frac{x}{2}}}\mathop {\lim }\limits_{x \to {0^ - }} \left( {a\cos \frac{x}{2} - 2\sin \frac{x}{2}} \right) = a\end{array}$

Để tồn tại $f'\left( 0 \right) \Leftrightarrow \mathop {\lim }\limits_{x \to {0^ + }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to {0^ - }} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} \Leftrightarrow a = 1.$

Chọn A.

Phương pháp giải:

+) Tính đạo hàm của hàm số khi $x > 1$

+) Tính đạo hàm của hàm số khi $x < 1$

+) Sử dụng định nghĩa đạo hàm, xét sự tồn tại của đạo hàm của hàm số tại x = 1.

Lời giải chi tiết:

Với $x > 1$ ta có: $f\left( x \right) = {x^2} - 3x + 1 \Rightarrow f'\left( x \right) = 2x - 3$

Với $x < 1$ ta có : $f\left( x \right) = 2x + 2 \Leftrightarrow f'\left( x \right) = 2$

Với x = 1 ta có : $\mathop {\lim }\limits_{x \to {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ + }} \left( {{x^2} - 3x + 1} \right) =  - 1 \ne f\left( 1 \right) = 4 \Rightarrow$ Hàm số không liên tục tại $x = 1,$  do đó không có đạo hàm tại $x = 1.$

Vậy $f'\left( x \right) = \left\{ \begin{array}{l}2x - 3\,\,\,khi\,\,x > 1\\2\,\,\,\,\,\,\,\,\,\,\,\,\,khi\,\,x < 1\end{array} \right.$

Chọn B.

Phương pháp giải:

- Tìm $a$ để hàm số liên tục tại $x = 0$.

- Với giá trị $a$ vừa tìm được, tính $f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}$, xét xem đạo hàm có tồn tại hay không (giới hạn có hữu hạn hay không).

Lời giải chi tiết:

TXĐ: $D = \left( { - \infty ;1} \right],\,\,x = 0 \in D$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to 0} f\left( x \right) = \mathop {\lim }\limits_{x \to 0} \frac{{\sqrt {1 - x}  - 1}}{x} = \mathop {\lim }\limits_{x \to 0} \frac{{ - x}}{{x\left( {\sqrt {1 - x}  + 1} \right)}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \frac{{ - 1}}{{\sqrt {1 - x}  + 1}} = \frac{{ - 1}}{2}\\f\left( 0 \right) = a\end{array}$

Để hàm số liên tục tại $x = 0$ thì $\mathop {\lim }\limits_{x \to 0} f\left( x \right) = f\left( 0 \right) \Leftrightarrow a =  - \frac{1}{2}$.

Khi đó ta có: $f\left( x \right) = \left\{ \begin{array}{l}\frac{{\sqrt {1 - x}  - 1}}{x}\,\,khi\,\,x \ne 0,\,\,x \le 1\\\,\,\,\,\,\, - \frac{1}{2}\,\,\,\,\,\,\,\,\,khi\,\,x = 0\end{array} \right.$.

$\begin{array}{l} \Rightarrow f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{{\sqrt {1 - x} - 1}}{x} + \frac{1}{2}}}{x} = \mathop {\lim }\limits_{x \to 0} \frac{{2\sqrt {1 - x} - 2 + x}}{{2{x^2}}}\\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \frac{{4\left( {1 - x} \right) - {{\left( {2 - x} \right)}^2}}}{{2{x^2}\left( {2\sqrt {1 - x} + 2 - x} \right)}}\\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \frac{{ - {x^2}}}{{2{x^2}\left( {2\sqrt {1 - x} + 2 - x} \right)}}\\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \frac{{ - 1}}{{2\left( {2\sqrt {1 - x} + 2 - x} \right)}} = \frac{{ - 1}}{8} \end{array}$

Vậy để hàm số có đạo hàm tại $x = 0$ thì $a =  - \frac{1}{2}$.

Chọn A.

Phương pháp giải:

- Tìm $a$ để hàm số liên tục tại $x =  - 1$, từ đó rút $b$ theo $a$.

- Tính $f'\left( { - {1^ + }} \right) = \mathop {\lim }\limits_{x \to  - {1^ + }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}}$, $f'\left( { - {1^ - }} \right) = \mathop {\lim }\limits_{x \to  - {1^ - }} \frac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}}$ .

- Để hàm số tồn tại đạo hàm tại $x =  - 1$ thì $f'\left( { - {1^ + }} \right) = f'\left( { - {1^ - }} \right)$.

Lời giải chi tiết:

TXĐ: $D = \mathbb{R},\,\,x =  - 1 \in D$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to  - {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {1^ + }} \left( { - {x^2} + a} \right) = a - 1 = f\left( { - 1} \right)\\\mathop {\lim }\limits_{x \to  - {1^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {1^ - }} \left( {{x^2} + bx} \right) = 1 - b\end{array}$

Để hàm số liên tục tại $x =  - 1$ thì $\mathop {\lim }\limits_{x \to  - {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {1^ - }} f\left( x \right) = f\left( { - 1} \right)$$\Leftrightarrow a - 1 = 1 - b \Leftrightarrow a + b = 2$.

Tính đạo hàm tại điểm $x =  - 1$:

$\begin{array}{l}f'\left( { - {1^ + }} \right) = \mathop {\lim }\limits_{x \to  - {1^ + }} \frac{{ - {x^2} + a - \left( { - 1 + a} \right)}}{{x + 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to  - {1^ + }} \frac{{ - {x^2} + 1}}{{x + 1}} = \mathop {\lim }\limits_{x \to  - {1^ + }} \left( { - x + 1} \right) = 2\\f'\left( { - {1^ - }} \right) = \mathop {\lim }\limits_{x \to  - {1^ - }} \frac{{{x^2} + bx - \left( { - 1 + a} \right)}}{{x + 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to  - {1^ - }} \frac{{{x^2} + bx + 1 - a}}{{x + 1}}\end{array}$

Thay $a = 2 - b$ ta có:

$\begin{array}{l}f'\left( { - {1^ - }} \right) = \mathop {\lim }\limits_{x \to  - {1^ - }} \frac{{{x^2} + bx - 1 + b}}{{x + 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to  - {1^ - }} \frac{{\left( {x - 1} \right)\left( {x + 1} \right) + b\left( {x + 1} \right)}}{{x + 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to  - {1^ - }} \left( {x - 1 + b} \right) = b - 2\end{array}$.

Để hàm số có đạo hàm tại $x =  - 1$ thì $f'\left( { - {1^ + }} \right) = f'\left( { - {1^ - }} \right)$$\Leftrightarrow b - 2 = 2 \Leftrightarrow b = 4$$\Rightarrow a = -2$.

Vậy $a = -2,\,\,b = 4$.

Chọn B.

Phương pháp giải:

$I\left( 4 \right) = Q'\left( 4 \right) = \mathop {\lim }\limits_{t \to 4} \frac{{Q\left( t \right) - Q\left( 4 \right)}}{{t - 4}}$

Lời giải chi tiết:

$I\left( 4 \right) = Q'\left( 4 \right) = \mathop {\lim }\limits_{t \to 4} \frac{{Q\left( t \right) - Q\left( 4 \right)}}{{t - 4}}$.

       $= \mathop {\lim }\limits_{t \to 4} \frac{{2{t^2} + t - 36}}{{t - 4}} = \mathop {\lim }\limits_{t \to 4} \frac{{\left( {t - 4} \right)\left( {2t + 9} \right)}}{{t - 4}} = \mathop {\lim }\limits_{t \to 4} \left( {2t + 9} \right) = 17\,\,\left( A \right)$.

Chọn C.

Phương pháp giải:

- Tìm điều kiện để hàm số liên tục tại $x = 0$: $\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = f\left( 0 \right)$.

- Tìm điều kiện để hàm số có đạo hàm tại $x = 0$: $f'\left( {{0^ + }} \right) = f'\left( {{0^ - }} \right)$, với $f\left( {{0^ \pm }} \right) = \mathop {\lim }\limits_{x \to {0^ \pm }} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}$.

Lời giải chi tiết:

TXĐ: $D = \mathbb{R},\,\,x = 0 \in D$.

Để hàm số có đạo hàm tại ${x_0} = 0$, trước hết hàm số phải liên tục tại $x = 0$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ + }} \left( {a{x^2} + bx + 1} \right) = 1 = f\left( 0 \right)\\\mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} \left( {ax - b - 1} \right) =  - b - 1\end{array}$

Để hàm số liên tục tại $x = 0$ thì $\mathop {\lim }\limits_{x \to {0^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {0^ - }} f\left( x \right) = f\left( 0 \right)$ $\Leftrightarrow  - b - 1 = 1 \Leftrightarrow b =  - 2$.

Ta có:

$\begin{array}{l}f'\left( {{0^ + }} \right) = \mathop {\lim }\limits_{x \to {0^ + }} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {0^ + }} \dfrac{{a{x^2} + bx + 1 - 1}}{x}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {0^ + }} \left( {ax + b} \right) = b =  - 2\\f'\left( {{0^ - }} \right) = \mathop {\lim }\limits_{x \to {0^ - }} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {0^ - }} \dfrac{{ax - b - 1 - 1}}{x}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {0^ - }} \dfrac{{ax}}{x} = a\end{array}$

Để hàm số có đạo hàm tại $x = 0$ thì $f'\left( {{0^ + }} \right) = f'\left( {{0^ - }} \right)$ $\Leftrightarrow a =  - 2$.

Vậy $T = a - 2b =  - 2 - 2.\left( { - 2} \right) = 2$.

Chọn C.

Phương pháp giải:

Sử dụng công thức tính số gia của hàm số $y = f\left( x \right)$ tại điểm $x = {x_0}$ là: $\Delta y = f\left( {{x_0} + \Delta x} \right) - f\left( {{x_0}} \right)$.

Lời giải chi tiết:

$\begin{array}{l}\Delta y = f\left( {1 + \Delta x} \right) - f\left( 1 \right)\\\,\,\,\,\,\,\, = {\left( {1 + \Delta x} \right)^2} + 2\left( {1 + \Delta x} \right) - {1^2} - 2.1\\\,\,\,\,\,\,\, = {\left( {\Delta x} \right)^2} + 2\Delta x + 1 + 2 + 2\Delta x - 3\\\,\,\,\,\,\,\, = {\Delta ^2}x + 4\Delta x\end{array}$

Chọn B.

Phương pháp giải:

- Tìm điều kiện để hàm số liên tục tại $x = 1$.

- Tính $f'\left( {{1^ \pm }} \right) = \mathop {\lim }\limits_{x \to {1^ \pm }} \dfrac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}$.

- Để hàm số có đạo hàm tại $x = 1$ thì $f'\left( {{1^ + }} \right) = f'\left( {{1^ - }} \right)$.

Lời giải chi tiết:

TXĐ: $D = \mathbb{R}$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ + }} \left( {a{x^3} + 2{x^2} + 3bx + 2} \right) = a + 3b + 4\\\mathop {\lim }\limits_{x \to {1^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ - }} \left( {\sqrt {5 - 4x}  - 2x} \right) =  - 1 = f\left( 1 \right)\end{array}$

Để hàm số có đại hàm tại $x = 1$ thì hàm số phải liên tục tại $x = 1$$\Rightarrow a + 3b + 4 =  - 1 \Leftrightarrow a + 3b =  - 5\,\,\,\left( 1 \right)$.

Ta có:

$\begin{array}{l}f'\left( {{1^ - }} \right) = \mathop {\lim }\limits_{x \to {1^ - }} \dfrac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ - }} \dfrac{{\sqrt {5 - 4x}  - 2x + 1}}{{x - 1}} =  - 4\\f'\left( {{1^ + }} \right) = \mathop {\lim }\limits_{x \to {1^ + }} \dfrac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ + }} \dfrac{{a{x^3} + 2{x^2} + 3bx + 2 + 1}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ + }} \dfrac{{\left( { - 5 - 3b} \right){x^3} + 2{x^2} + 3bx + 3}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ + }} \dfrac{{\left( { - 5 - 3b} \right){x^3} + 2{x^2} - 2 + 3bx - 3b + 3b + 5}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ + }} \dfrac{{\left( { - 5 - 3b} \right)\left( {{x^3} - 1} \right) + 2\left( {x - 1} \right)\left( {x + 1} \right) + 3b\left( {x - 1} \right)}}{{x - 1}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to {1^ + }} \left[ {\left( { - 5 - 3b} \right)\left( {{x^2} + x + 1} \right) + 2\left( {x + 1} \right) + 3b} \right]\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 3\left( { - 5 - 3b} \right) + 4 + 3b =  - 6b - 11\end{array}$

Để hàm số có đạo hàm tại $x = 1$ thì $f'\left( {{1^ + }} \right) = f'\left( {{1^ - }} \right)$$\Leftrightarrow  - 6b - 11 =  - 4 \Leftrightarrow b = \dfrac{{ - 7}}{6}$.

Thay vào (1) ta có: $a + 3.\dfrac{{ - 7}}{6} =  - 5 \Leftrightarrow a =  - \dfrac{3}{2}$

Vậy $ab =  - \dfrac{3}{2}.\dfrac{{ - 7}}{6} = \dfrac{7}{4}$.

Chọn C.

Phương pháp giải:

- Xét tính liên tục của hàm số tại $x =  - 1$.

- Tính $f'\left( 1 \right) = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}}$.

Lời giải chi tiết:

Ta có: $\mathop {\lim }\limits_{x \to  - 1} f\left( x \right) = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{\sqrt {{x^3} + 2{x^2} + x + 4} {\rm{\;}} - 2}}{{x + 1}}$.

                                $\begin{array}{l} = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{{x^3} + 2{x^2} + x + 4 - 4}}{{\left( {x + 1} \right)\left( {\sqrt {{x^3} + 2{x^2} + x + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{x{{\left( {x + 1} \right)}^2}}}{{\left( {x + 1} \right)\left( {\sqrt {{x^3} + 2{x^2} + x + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{x\left( {x + 1} \right)}}{{\sqrt {{x^3} + 2{x^2} + x + 4}  + 2}} = 0 = f\left( { - 1} \right)\end{array}$

$\Rightarrow$ Hàm số đã cho liên tục tại $x =  - 1$.

Ta có:

$f'\left( 1 \right) = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{f\left( x \right) - f\left( { - 1} \right)}}{{x + 1}} = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{\sqrt {{x^3} + 2{x^2} + x + 4} {\rm{\;}} - 2}}{{{{\left( {x + 1} \right)}^2}}}$

            $\begin{array}{l} = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{{x^3} + 2{x^2} + x + 4 - 4}}{{{{\left( {x + 1} \right)}^2}\left( {\sqrt {{x^3} + 2{x^2} + x + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to  - 1} \dfrac{{x{{\left( {x + 1} \right)}^2}}}{{{{\left( {x + 1} \right)}^2}\left( {\sqrt {{x^3} + 2{x^2} + x + 4}  + 2} \right)}}\\ = \mathop {\lim }\limits_{x \to  - 1} \dfrac{x}{{\sqrt {{x^3} + 2{x^2} + x + 4}  + 2}} = \dfrac{{ - 1}}{4}\end{array}$

Chọn A.

Phương pháp giải:

- Xét tính liên tục của hàm số tại $x = 0$.

- Tính giới hạn $\mathop {\lim }\limits_{x \to 0} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}$. Nếu giới hạn này tồn tại thì $f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}}$.

Lời giải chi tiết:

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to 0} f\left( x \right) = \mathop {\lim }\limits_{x \to 0} \dfrac{{2 - \sqrt {4 - x} }}{x} = \mathop {\lim }\limits_{x \to 0} \dfrac{{4 - 4 + x}}{{x\left( {2 + \sqrt {4 - x} } \right)}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \dfrac{1}{{2 + \sqrt {4 - x} }} = \dfrac{1}{{2 + 2}} = \dfrac{1}{4}\\ \Rightarrow \mathop {\lim }\limits_{x \to 0} f\left( x \right) = f\left( 0 \right)\end{array}$

$\Rightarrow$ Hàm số đã cho liên tục tại $x = 0$.

Ta có:

$\begin{array}{l}\mathop {\lim }\limits_{x \to 0} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \mathop {\lim }\limits_{x \to 0} \dfrac{{\dfrac{{2 - \sqrt {4 - x} }}{x} - \dfrac{1}{4}}}{x}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \dfrac{{8 - 4\sqrt {4 - x}  - x}}{{4{x^2}}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \dfrac{{{{\left( {8 - x} \right)}^2} - 16\left( {4 - x} \right)}}{{4{x^2}\left( {8 - x + 4\sqrt {4 - x} } \right)}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \dfrac{{{x^2} - 16x + 64 - 64 + 16x}}{{4{x^2}\left( {8 - x + 4\sqrt {4 - x} } \right)}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \mathop {\lim }\limits_{x \to 0} \dfrac{1}{{4\left( {8 - x + 4\sqrt {4 - x} } \right)}}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \dfrac{1}{{4\left( {8 - 0 + 4.2} \right)}} = \dfrac{1}{{64}}\end{array}$ 

Vậy $f'\left( 0 \right) = \mathop {\lim }\limits_{x \to 0} \dfrac{{f\left( x \right) - f\left( 0 \right)}}{{x - 0}} = \dfrac{1}{{64}}$.

Chọn C.